4.5
Diasporic Experience and the Need for Topological Methods
Historically, architecture has struggled with how to represent movement, as it inevitably also encapsulates time, and architectural representation has traditionally remained static.1 However, in order to account for movement within architecture, it is not simply a question of being able to represent dynamic situations, it is also the very nature of architectural space, conceptualised through the constraints of Euclidean geometry, that needs to be questioned. Although space as a concept has exploded in cultural theory, geography and many other disciplines, including also architectural theory, in the practice of architecture it remains a container, bounded and secure in its three dimensions. Movement provokes a crisis in this containerised space.
Movement can, of course, occur at different scales – the movement of populations across borders, or the movement of a person across a city street. Often, these two scales collide: a migrant body moving through a street in a European city negotiates both these scales at once, a space that is impossible to define in standard Euclidean terms. As space of this kind cannot readily be represented, the inhabitations that create such spatial conditions remain hidden to the traditional gaze of the architect. Yet, it is not only the (in)visibility of these spaces that is important, but also their operation. Space of this kind needs to be conceptualised as the product of particular sets of relations to notions of scale, time and belonging. It is, therefore, directly related to the production of subjectivities and the often precarious and ambivalent nature of migrant lives. In order to operate with what I shall call migrant space, an approach is required that can switch scales and emphasis, from the intimate to the institutional, from the local to the global, and from systems to bodies.
This switching of scale is also related to the way in which change is conceptualised in contemporary society. Whereas traditionally society was considered mostly static, change being thought of as extensive and atypical, in the recent ‘topological turn’ in social theory, change is considered normal and immanent.2 This requires a shift in the way cities are understood. If change and movement are the norm, then the switching of scales and the different modes of belonging that migrants embody must also be understood as part of the course. Multiple and often conflicting subjectivities that encompass staccato modes of belonging, loyalties that are (dis)loyal, the foldedness of time that results from mixing nostalgias with contemporary trans-local connections, are becoming more prevalent in all our lives. For migrants and diasporas, these conditions are the result of displacement, but, in a globalised world, such responses do not necessarily require physical displacement. In fact, such modes of inhabiting are typical of a topological tendency in contemporary culture that not only reveals itself in situations relating to notions of home and belonging, but in many other aspects of our social lives.3
In the context of migration, these effects reveal themselves most forcefully in the different ways that notions of belonging, of inclusion and exclusion are affected. The ease with which relations can be made across large distances, yet are fragmented in topographic proximity, is a classic example. These trans-local connections described by Appadurai not only create relations, they also fragment them.4 Often, this fragmentation is only described at the level of social integration, but it is also related to the way in which states are functioning within Europe. On the one hand, economically driven processes are creating pockets of ‘the global inside the national’, through processes of denationalisation;5 on the other hand, the very system upon which modern Europe was constructed is fragmenting. The idea of a contiguous European territory, defined through the Westphalian system of states, was built precisely to create a static and peaceful order, resting upon the idea of inclusion and exclusion as binary metrics and on a notional equality of all states.6 With the power of global capital and the unevenness of development, this idea of equality is being systematically eroded. The faltering of the Westphalian project can, therefore, be related to the new ways in which Europe is being reconstituted through new relations, less dependent on proximity, which also have a profound effect on its diasporic populations.
Topology, surface and mediation
Such phenomena require different methods of analysis and intervention, a new understanding of the social. If change is thought of as immanent, we require an approach that is recursive and iterative. Practices of mediation and affect take on important functions, as they are able to negotiate a shifting terrain. Such practices are related to the changing role of the surface in contemporary society,7 where change occurs quickly and is reacted to almost immediately. As Adkins and Lury state, this move to the surface had already occurred in the 1920s in the sociologist Siegfried Kracauer’s description of modern society. In his essay The Mass Ornament, Kracauer describes the appearance of masses through a reading of the Tiller Girls8 as ornamental phenomena. ‘The ornament, detached from its bearers, must be understood rationally. It consists of lines and circles like those found in text books on Euclidean geometry.’9 It perhaps comes as no surprise that Kracauer was trained as an architect. This reductive description of the surface through recourse to three fixed co-ordinates chimed with the description of a mass society where the Tiller Girls became a ‘fraction of a figure’,10 and the audience became spectator. If, in modern society, the surface was Euclidean, Adkins and Lury ask what model of the surface is required in contemporary society. Here, the surface becomes folded and describes a space that is non-Euclidean. For architecture too, the modern project has been a ‘turn to the surface’ that foregrounds questions of mediation, such as the mediation of architecture through the machine, functionality, etc. Adkins and Lury’s question is pertinent for us as well. In this model of a complex and non-linear society, on what type of surface is mediation taking place? The concept of the surface is fraught in architecture; on the one hand, it conjures images of shiny buildings and literally folded and undulating surfaces of installations and plazas. On the other hand, the surface as metaphor is the place where social relations occur. However, following Lury, how can the surface also be thought of as method? The topological tendency described above as the changing role of the surface is simultaneously a concept and a mode of enquiry. In the practice of architecture, it translates into methods that can both represent and intervene in the spatial.
These are topological methods. Topology is a branch of modern mathematics, the study of spaces ‘reduced’ to surfaces.11 It is the study of continuity and connectivity through continuous deformations. In other words, topology is concerned with those instances where change and movement do not break connections and relations. Topology has several branches, but the most general is point-set theory, which studies the properties of topological spaces. It is still a contested area, where much of the argument centres around the problem of the continuum, first defined by Georg Cantor as: ‘How many points are there in a straight line . . .’ or ‘How many different sets of integers do there exist?’12 Different approaches to point-set theory provide very different attitudes to this as yet unanswerable question. For some, this is a question of connectedness, or the openness of a set; for others, it is the constitution of the set itself that is problematic.13 For the mathematician Brian Rotman, categories and arrows are more useful than sets, as they are inherently relational, and what is included in a category is defined by external relations. There is no originary or primordial belonging to a set, even an open one, as was the case with the former viewpoint. The definition of such an inclusion is, for Rotman, a diagrammatic problem that must be approached through vectors: ‘in contrast to the fixity of sets and the membership relation, arrows and composition connote movement or transformation’.14
Movement is inherent in topology, described as categories and relations. But, even in thinking of the topological through sets and memberships, what remains is a very different approach to the idea of measurement. As Sha writes: ‘Topology provides an anexact (in Deleuze’s sense) mode of articulation, that does not need numerical measure, equations, exact data, statistics.’15 It is this relation to measuring, a precision that is allowed to remain fuzzy, that is so useful for a way of thinking of space as movement in architecture. As Lury states, in topology, the two properties conflated in Euclidean mathematics, order and value, are brought together in relation to each other:
In the topological thinking of multiplicity, however, ordering and value are brought together without reference to an external measure, but rather by – or in – relations in which the performative capacities of number to order and value are locally combined in different ways to produce spaces more general than those described by Euclid.16
What this way of thinking highlights is how value is created. In terms of architecture and urban practice, it asks whose experience is counted and in what way, and what role do devices and instruments have in this counting and measuring? Here, the question reverts to that of the surface. This move to general spaces that may be combined locally but could also have other, more far-flung, connections is exactly the relation of the folded surface, where intensities create moments of opportunity and where movement is the norm.17
What might these general spaces entail for architecture? They point to a way of describing space as viewed from different angles and from different and overlapping points of view. They hint at a way of engaging spaces as having differing values and differing temporalities, of conceptualising spaces and their inhabitations as having the capacity to change. In order to intervene in such spaces, a mode of representation is required that can account for these different perspectives, but, crucially, can also make the conditions for relations between them emerge or be proposed. Thus, a central quality of a topological methodology in architecture would be mediation.
Affective methods and the space of possibilities
Returning to the ‘impossible’ space defined by the migrant body moving through the city, it is the body’s surface that describes this space. Brian Massumi claims affect entails a different language to bodies that cannot be expressed in words: ‘For affect is synesthetic, implying a participation of the senses in each other: the measure of a living thing’s potential interactions is its ability to transform the effects of one sensory mode into those of another’.18 The response of bodies is, therefore, not predetermined but unfolds in time, relationally. In topological terms, this unfolding occurs as points along a line. Time is considered, not as the time taken for an event to happen, but the delay between receiving an affect and the reception of it, or reaction to it. Here, affect is a way of thinking dynamically about, not just bodies in space, but about space unfolding around bodies through their relations and encounters of speed and slowness. As architects, how might we represent this quality that bodies have of creating topological spaces of affect?

Figure 4.5.1 Stoke Newington High Street, London Borough of Hackney, 2007
Source: Photograph by Nishat Awan
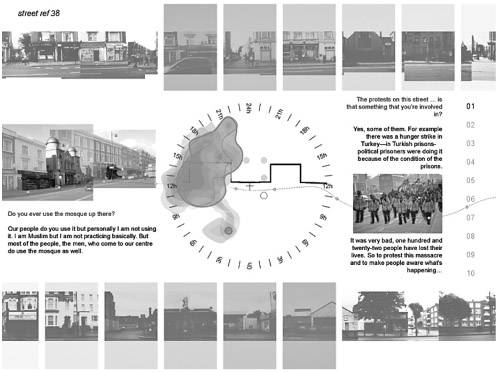
A project based in the London Borough of Hackney might give some clues. It used the body as a way of negotiating the affective terrain of a contested street,19 with many Turkish and Kurdish shops and businesses (Figure 4.5.1). It was a site of regular protest by Kurdish groups against events in Turkey and, therefore, a highly contested space, differently experienced by people from different backgrounds. The movement of bodies along the street, whether collectively in the form of a protest, or in the habitual exchanges of everyday life, created an invisible terrain of appropriated space that we attempted to map. This movement of the body was represented in a number of different ways: first, as experience, where walking with others was a way of documenting the space; second, as temporality, using information from our conversations that described how the street was occupied and used at different times; and finally, as an exchange between different spaces, from those that we physically occupy to the mental spaces that make connections elsewhere. This last aspect is perhaps the most important, the attempt to represent the fluid connections that we all make across different times and spaces, but that are greater in number and more intense in the case of diasporas. The maps took the information from the original walks (where we went, at what time, the connections made to other places) and encoded them into a three-dimensional point cloud, with the third dimension used for time. An artificial neural network (ANN) was then used to create a relational map of the street that represented each person’s walk as a ‘sphere’ or spatial envelope (Figure 4.5.2).20
ANNs are mathematical models that describe a topological space inspired by the structure and functional aspects of biological neural networks.21 Nodes and connections within a computational system process inputs and create outputs, a representation of the transmission of information through synapses and neurons in the brain. Usually, such programs are used to model complex relationships and patterns in datasets, typically being used for classification. There are a range of different types of neural network that vary in topological organisation and, consequently, in the transmission of data. For this project, a specific ANN derived from ‘the self-organising map’ (SOM) was used, which is typically a two-dimensional map of n-dimensional inputs.22 In cognitive and computational science, the SOM is used as a tool for categorisation, but we took a different approach, based on the observation that, just as in real life the relations between people, objects and spaces are altered as soon as something extra is added or someone else arrives, so the same is also true for a neural network, which is, in essence, a map of relations. It is solely a representation of the original inputs – there is no field upon which inputs are distributed, and, therefore, there can be no categories, only relations. The advantage of using ANNs lies, not in their ability to provide classifications of data, but in their ability to provide approximations of it. In fact, what are mapped are topological relationships, rather than topographic descriptions.
In topological terms, then, the ANN acted as a manifold and, combined with the walks, it resulted in an affective method for understanding the city as experience. The dynamic model allowed movement to be represented in overlapping ways and at different scales. It was able to encapsulate the physical movement along the street as a dynamic section, where elevations at the top and bottom of the screen were used as a navigational device (Figure 4.5.3). The specific effects that occur through the displacement of the migrant subject were represented by the green map in the middle, which was produced by the ANN and represented the amount of activity in a particular space over a period of time, as well as the relations made to other spaces. Finally, extracts of conversations and images from the walks were used to create a further layer of information. Using the ANN to represent diasporic space allowed a standard two-dimensional map of the walks to have experience folded back into it. The interface then brought the n-dimensional space of the manifold back into the standard Euclidean space of architectural representation. In this move from three- to n-dimensions and back again, the map looks very different. It is necessarily imprecise, it resists explanation, it suggests a move away from architecture and urban design as problem-solving towards the mediation of open-ended spatial possibilities that allow for multiple perspectives.
Notes
1 Traditional architectural representation has been confined to two dimensions, such as a plan or a section, or to the simulation of a third dimension, for example in perspective drawing. In order to encapsulate movement within this plane, techniques from other disciplines and practices have sometimes been borrowed: for example, from Futurist paintings or from the notation systems developed to draw the movement of a body through space in dance. More recently, the productive relationship with film and animation, has allowed a temporal dimension to be included. (For more on the relationship between architecture and representation, see Pérez-Gómez and Pelletier 2000).
2 Lash and Lury 2007. S. Lash (2009) Afterword: In praise of the a posteriori sociology and the empirical, European Journal of Social Theory, vol. 12, no. 1, pp. 175–87.
3 C. Lury, L. Parisi and T. Terranova (2012) Introduction: The becoming topological of culture, Theory, Culture & Society, vol. 29, no. 4–5, pp. 3–35.
4 A. Appadurai (1990) Disjuncture and difference in the global cultural economy, Public Culture, vol. 2, no. 2, pp. 1–23.
5 S. Sassen (2010) The global inside the national: A research agenda for sociology, Sociopedia.isa. Available at: www.saskiasassen.com/pdfs/publications/the-global-inside-the-national.pdf (accessed 18 August 2014).
6 D.E. Davis (1999) ‘Chaos and governance’, in Political Power and Social Theory, vol. 13.
7 L. Adkins and C. Lury (2009) Introduction: What is the empirical? European Journal of Social Theory, vol. 12, no. 1, pp. 5–20.
8 A dancing troupe formed by John Tiller that originated the precision dancing technique. Kracauer was fascinated by the dissolving of individuals into one mass unit by the linking of arms and through a militarised aesthetic.
10 Ibid., p. 76.
11 Here, I use ‘reduced’ as Deleuze would use it in his description of the fold: ‘The simplest way of stating the point is by saying that to unfold is to increase, to grow; whereas to fold is to diminish, to reduce, “to withdraw into the recesses of a world”’ (Deleuze 1993, pp. 8–9). This is not a reduction in the colloquial sense, but a reduction that leads to an intensity.
12 K. Godel (1947) What is Cantor’s continuum problem? The American Mathematical Monthly, vol. 54, no. 9, pp. 515–25, p. 515.
13 X.W. Sha (2012) Topology and morphogenesis, Theory, Culture and Society, vol. 29, no. 4–5, pp. 220–46.
14 Rotman 2012, p. 254.
15 Sha 2012, op. cit., pp. 222–3.
16 C. Lury (2009) ‘From one to multiplicity’, in Ascione et al. 2009, p. 80.
17 Deleuze 1993.
18 Massumi 2002, p. 35.
19 The project was carried out in collaboration with computational designer Phil Langley and as part of PhD research at University of Sheffield, funded by AHRC. For more detailed information on the project, see N. Awan and P. Langley (2013) Mapping migrant territories as topological deformations of space, Space and Culture, vol. 16, no. 1.
20 Sloterdijk and Hoban 2011.
21 In the brain, neurons receive signals through synapses located on the membrane of the neuron. When the signal received is strong enough, the neuron is activated and emits a signal, which can be passed on to another synapse and, if strong enough, can activate further neurons. ANNs are simplified and highly abstracted versions of this process that consist of an input and an output, with the strength of the signal being determined by a multiplier. They are used in a wide variety of settings for their ability to simulate complex, non-linear relationships.
22 Kohonen 2001.